Cost of Borrowing
The cost of borrowing is a simple concept. If there are no costs associated with your mortgage other than the interest rate, then the cost of borrowing is the annual interest rate. Without getting into legalese, government legislation or which costs are applied against a mortgage let us look at the mathematical concept in its simplicity. The cost of borrowing is the same calculation as if the mortgage was being discounted. The total of all the costs is nothing more than the discount off the face value of the mortgage. Canadian Mortgage Brokers use the “semi-annual” compounding button, while American Mortgage Brokers use the “Monthly” compounding button.
If you borrow $100,000 mortgage on Monday morning at your local bank and then in the afternoon pay out a total of $2500 in fees to a mortgage broker, then in reality you are receiving a net amount of $97,500 on Monday but your banker is obviously asking for monthly payments based upon $100,000.
If an investor wanted to buy your 6% mortgage of $100,000 on Monday and only wanted to pay $97,500 so that he could achieve a higher rate than 6%, the calculation of the higher interest rate (aka “yield”) is the same calculation as the cost of borrowing calculation.
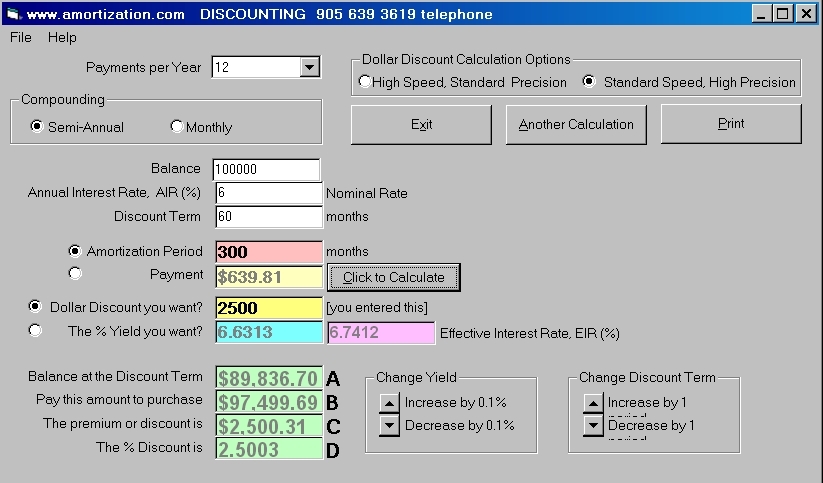
To do the cost of borrowing calculation, using the discount module, the total costs of $2500 is entered into the yellow input box, by first clicking on the radio dial, then clicking on the “Click to Calculate” button. To comply with the new Ontario legislation (January 1, 2009) the cost of borrowing is 6.6313%. In practical terms the cost of borrowing is 6.63% at two decimal places.
NOTE: Prior to January 1, 2009 the Ontario government would have required a mortgage broker, in this example, to quote the EIR of 6.7412% as the cost of borrowing.
The new legislation of January 1,2009 also specifies another new definition of APR. When I figure out how the new APR calculation is done I will post it on this web site.
To do a Discount calculation, again using the same discount module, the new lender might have said that he wanted a rate of 6.6313% if he bought your mortgage. The requested rate is entered into the blue input box by pressing the radio dial in front of “The % yield you want?” prompt.
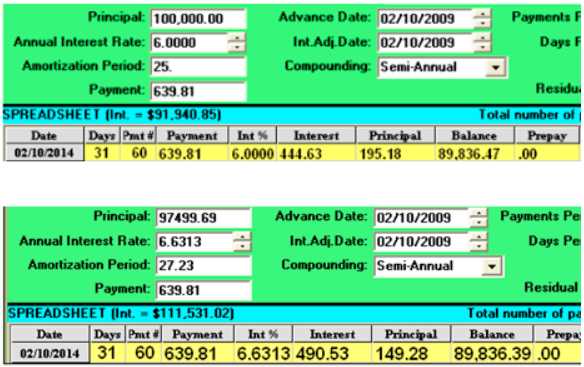
The investors yield (the annual interest rate he asked for) of 6.6313% gives rise to the same outstanding balance at the end of 60 months (within 8 cents). Don’t be alarmed but, it is almost impossible to get the outstanding balances at 60 months to be EXACT (even compared to “A”) because the balances are very sensitive to the number of decimal places in the interest rates and the rounding off of 60 separate calculations.
The investor pays $97,499.69 for your mortgage, for 60 monthly payments to him of $639.81 which will give the investor a 6.6313% yield. In lay terms, he lent you $97,499.69 on a 60 month term at a rate of 6.6313% and the balance owing after the 60th payment is $89,836.70 Most people will not quibble over 20 or 30 cents difference on a hundred thousand dollar transaction.
The reason the “Pay this amount to purchase” value in the Discount screen is 31 cents less than $97,500 is because the discounting program takes a guess at what the interest rate should and then iterates until it gets close to the actual value. It could be made to guess in smaller increments but it would slow down the calculation tremendously. It is still much faster than going through 17 time consuming steps on a financial calculator to arrive at the same answer. But once the Discount module has the info on the screen any number of fast “what if” scenarios can be instantly calculated at the click of a button, saving you a lot of time!
