Let us assume you purchased your house for $100,000 and sold it 6 years later to Bob for $200,000!

What is your return on your investment? Assuming you did not spend one penny to renovate or fix up the house, then your profit is $100,000 because you doubled your money in the six years. Growth upon growth is called compounding whether it is interest on an unpaid loan, growth of an investment or describing a bacteria culture growing in a petri dish. In this example your return on investment is 12.25%. This example was very real in Ontario from 1960 to 1990 as it described the average Real Estate markets growth. Real Estate was growing at approximately 12 % per year and therefore your home was doubling in value every six years.

You could also have elected to deposit $100,000 into a financial institution that paid you 12.25% each year and not touched any of the money for 6 years. The mathematics is exactly the same. Anyone that understands present value future value calculations understands the algebraic formula;

FV = PV (1+i)^n

“FV” is the future value
“PV” is the present value
“i” is the interest or growth per period
“n” is the number of periods of time

FV=100,000x(1.1225)^6
FV=200,000

The “^ n” means that the numbers in the brackets are raised to the nth power. If your algebra is rusty don’t fret, here is the example in a simpler format showing the growth upon growth;

12.25% interest (or growth) on $100 at the end of a year is $12.25 Add that to the $100 for a total of $112.25 or the same thing can be arrived at by multiplying the $100 by 1.1225

Thus for 6 years of compounded growth;
FV = 100,000 x 1.1225 x 1.1225 x 1.1225 x 1.1225 x 1.1225 x 1.1225
FV = 200,000

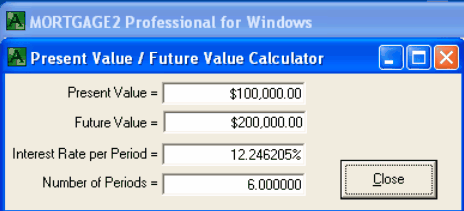
The 12.25% is called the annual compounded rate of growth (or compounded return) or simply the compounded rate of growth because the year is generally the period of time for comparing numbers in financial circles. To verify the 12.25% compounded rate one needs to use the Present Value/Future Value selection in the UTILITIES Menu of the MORTGAE2 PRO software that allows any three of the values in that algebraic formula to be entered and thus calculate the missing information, 12.246205%. If one is about to purchase a financial calculator exactly for this type of calculation I would suggest that you shop around carefully as not all calculators have this 3 out of 4 feature as an easy option. The MORTGAGE2PRO utility will allow the $100,000, $200,000 and the 6 years to be entered and then you easily click and calculate the 12.246205% compounded yearly rate.